I put the configurations I showed on the projector online:
You may move the points around to see how the configuration changes.
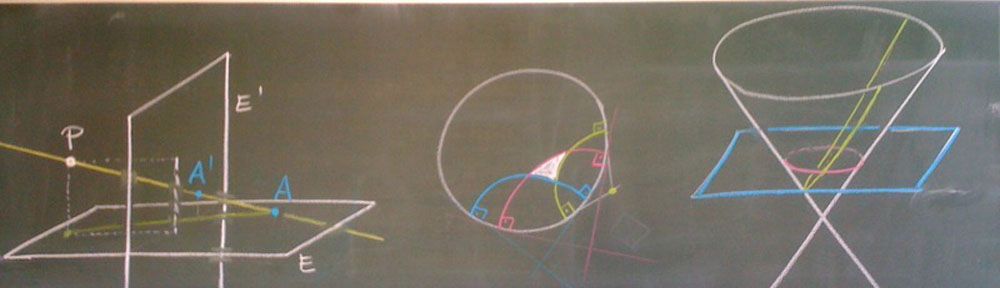
I put the configurations I showed on the projector online:
You may move the points around to see how the configuration changes.
Definition: Two triangles $\triangle_1 = \triangle(A_1, B_1, C_1)$ and $\triangle_2 = \triangle(A_2, B_2, C_2)$ are in perspective w.r.t. a point $S$ if
\[
S = (A_1 A_2) \cap (B_1 B_2) \cap (C_1 C_2)
\]
The triangles are in perspective w.r.t. a line $\ell$ if
\begin{align*}
A’ = (B_1 C_1) \cap (B_2 C_2)\\
B’ = (A_1 C_1) \cap (A_2 C_2)\\
C’ = (A_1 B_1) \cap (A_2 B_2)\\
\end{align*}
lie on $\ell$.
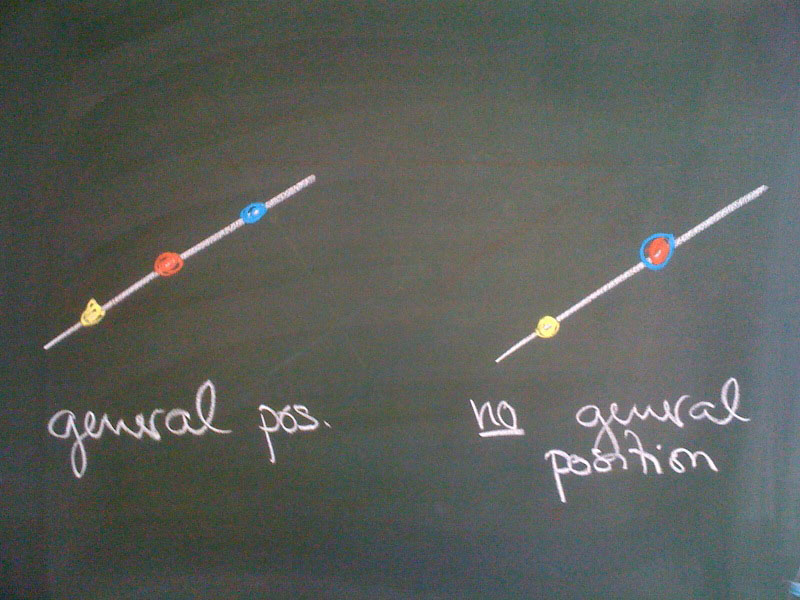
Definition. Let $P(V)$ be a projective space of dimension $n$. Then $n+2$ points in $P(V)$ are said to be in general position if no $n+1$ of them are contained in a $(n-1)$-dimensional projective subspace. In terms of linear algebra this implies that no $n+1$ representative vectors are linearly dependend, i.e. every $n+1$ are linearly independent.
Examples. If $n = 1$ we have to consider $n+2 = 3$ points on a projective line. These three points are in general position as long as they are disjoint.
Let us start with a general definition for an arbitrary projective space. In this lecture we will almost entirely deal with real projective spaces.
Definition. Let $V$ be a vector space over an arbitrary field $F$. The projective space $P(V)$ is the set of $1$-dimensional vector subspaces of $V$. If $\dim(V) = n+1$, then the dimension of the projective space is $n$.
If you don’t know how to use Latex, take a look at the source code of the lectures that already exist as blog entries, by pressing “edit” below an entry name in the “All Posts” overview. You will then see the latex source code that produces the formulas in the blog.
The following information also might be helpful for you.
The “Short Math Guide for Latex” by the AMS: ftp://ftp.ams.org/pub/tex/doc/amsmath/short-math-guide.pdf
A short introduction to Latex (in German): lkurzauf2
A wiki-books chapter about writing maths in Latex: http://en.wikibooks.org/wiki/LaTeX/Mathematics
Consider the linear projection map for the homogeneous coordinates of the central projection described in the first lecture. What are the images of the points on the line $l_\infty$ if we assign homogeneous coordinates $\svector{b_1\\b_2\\0}$ with $b_1 \neq 0$ to the points on the line?
How can you construct the perspective image of the first square given the position of the chessboard, your position (the “eye” point), and the position of the plane projected onto? The construction of the other “squares” is described in the first lecture.
The word geometry is of Greek origin ($\gamma\varepsilon\omega\mu\varepsilon\tau\rho\iota\alpha$) and means “measurement of the earth”. But the first accounts were found in Egypt and Mesopotamia (today Iraq) around 2000 BC. It was known how to calculate simple areas and volumes and people of that time already had an approximation of $\pi$. But no general statements/theorems and proofs were found. Around 600 BC in Greece, Thales was the first to deduce theorems from a set of axioms. At about 300 BC, Euclid wrote the book Elements in which he states the five axioms of what is today called Euclidean geometry. His fifth axioms is known as the parallel postulate and can be phrased as follows:
Given a line and a point not on this line, there is a unique line through the point parallel to the line.