Projective Geometry
Let us start with a general definition for an arbitrary projective space. In this lecture we will almost entirely deal with real projective spaces.
Definition. Let $V$ be a vector space over an arbitrary field $F$. The projective space $P(V)$ is the set of $1$-dimensional vector subspaces of $V$. If $\dim(V) = n+1$, then the dimension of the projective space is $n$.
- A $1$-dimensional projective space is a projective line.
- A $2$-dimensional projective space is a projective plane.
Canonical analytic description
Two vectors $v$ and $\tilde{v}$ span the same $1$-dimensional subspace if there exist $\lambda \neq 0$ such that $v = \lambda \tilde{v}$. This yields an equivalence relation on $V \setminus \{0\}$:
\[ v \sim \tilde{v}
:\Leftrightarrow \exists \lambda\neq0 : v = \lambda \tilde{v}.
\]
The points of the projective space $P(V)$ are equivalence classes of $\sim$:
\[P(V) = (V \setminus \{0\})/{\sim}.\]
We write $[v] \in P(V)$ and call $v$ a representative vector of the line $\{\lambda v \,|\, \lambda \in F\}$.
Models of real projective space
Let us have a look at different models for real projective space $\RP^2 = P(\R^3)$.
Subspace model
Consider all $1$-dimensional subspaces $\ell = \{\lambda v \,|\, v \in \R^3, v \neq 0, \lambda \in \R\}$ of $\R^n$.
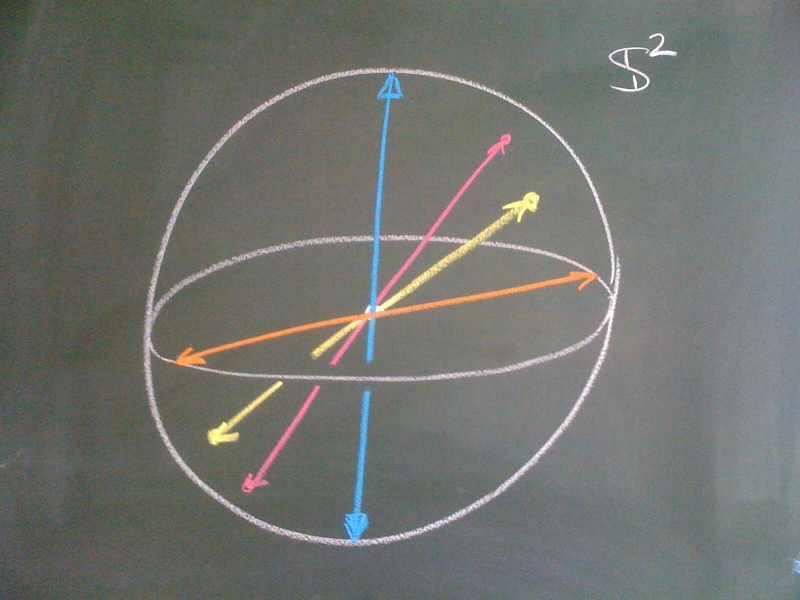
Sphere model
We may normalize non-zero vectors to become unit vectors. Then there are only two vectors representing the same line. If we now introduce an equivalence relation
\[
v \sim \tilde{v} \quad :\Leftrightarrow \quad
v = \pm\tilde{v} \text{ for $v,\tilde{v} \in \S^n$}
\]
we obtain $\RP^n = \S^n/\sim$.
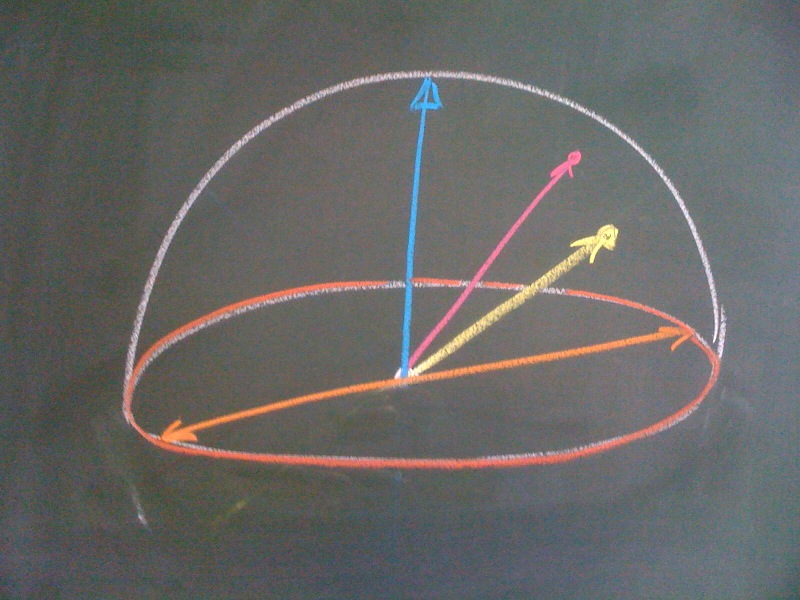
Hemisphere model
Consider the upper hemisphere $H = \left\{ \svector{x_1\\x_2\\x_3} \in \R^3 \,|\, \|x\| = 1, x_3 \geq 0 \right\}$. Then all non-horizontal lines have a unique representative vector in $H$ and we only need to identify points on the equator $H \cap \{ x \,|\, x_3 = 0 \}$.
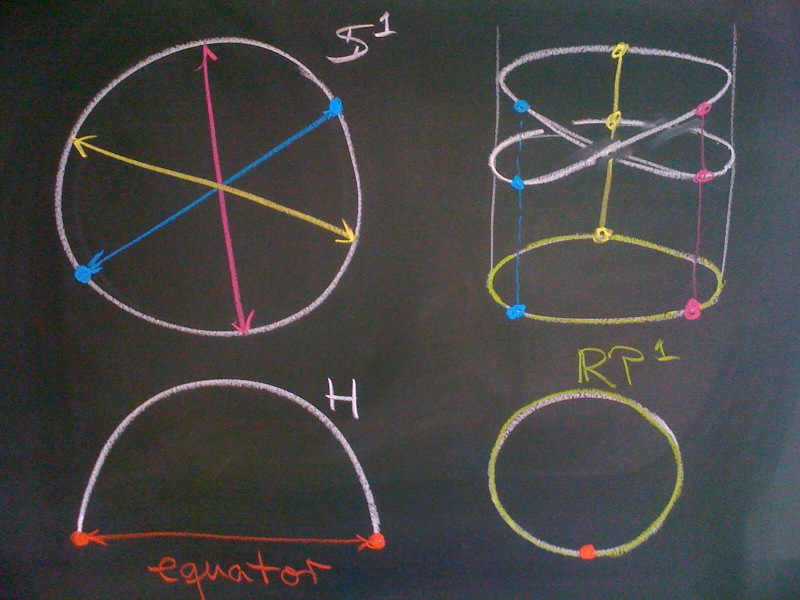
Examples of lower dimension:
- $\RP^0 = P(\R^1) = \S^0{/}{\sim} = \{*\}$ is just a single point.
- $\RP^1 = P(\R^2) = \S^1{/}{\sim} = \S^1$. This is most obvious using the hemisphere model, since the hemisphere is just a line and the two points on the equator are identified (bottom of the picture below). But we can also twist an $\S^1$ in a way such that opposite points come to lie on top of each other (top of picture below).
Homogeneous coordinates
Consider a basis $\{b_1, \ldots,b_{n+1}\}$ of the vector space $V$ and a vector $v\in V$, $v \neq 0$ with coordinates $(x_1,\ldots,x_{n+1})$, i.e. $v = \sum_{i=0}^{n+1} x_i b_i$. Then $(x_1,\ldots,x_{n+1})$ are homogeneous coordinates of $[v] \in P(V)$. These coordinates are not unique. They depend on the choice of basis and are then only unique up to a scalar factor:
\[
[v] = [x_1,\ldots,x_{n+1}] = [\lambda x_1, \ldots, \lambda x_{n+1}]
\text{, for $\lambda\neq 0$}.
\]
Homogeneous coordinates of $\RP^n$. Let $[v] \in \RP^n$ with coordinates $[v] = [x_1,\ldots,x_{n+1}]$. Since the homogeneous coordinates are only unique up to a scalar factor we do the following:
- if $x_{n+1} \neq 0$ then
\[[v] = [x_1,\ldots,x_{n+1}]=\left[\frac{x_1}{x_{n+1}},\ldots,\frac{x_n}{x_{n+1}},1\right]=[y_1,\ldots,y_{n+1}] \simeq\R^n.\]
The coordinates $(y_1,\ldots,y_{n+1})$ are affine coordinates for $[v]$ with $x_{n+1} \neq 0$. - if $x_{n+1} = 0$ then
\[ [v] = [x_1,\ldots,x_n,0] \simeq \RP^{n-1}.\]
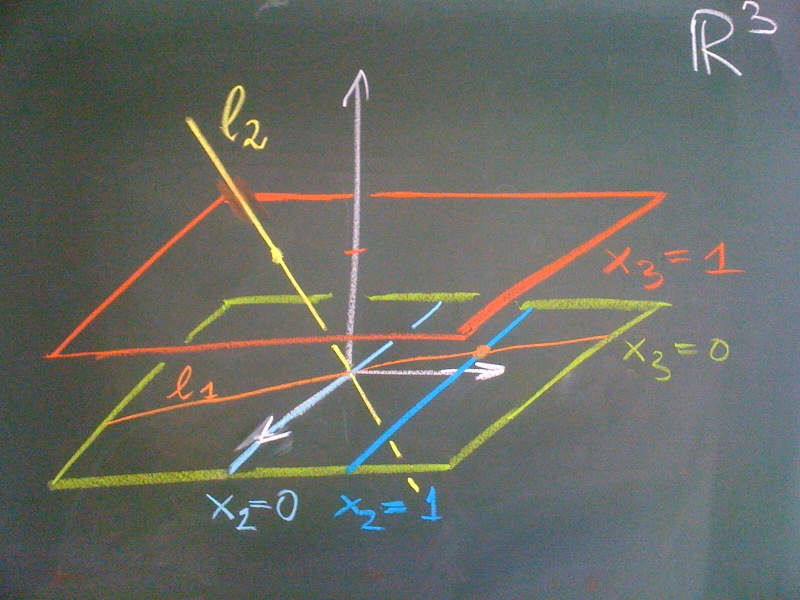
So we obtain the following decomposition $\RP^n = \R^n \cup \RP^{n-1}$. This can be iterated and $\RP^{n-1}$ may again be decomposed into $\R^{n-1}$ and $\RP^{n-2}$, and so on. The part isomorphic to $\R^n$ (e.g. $x_{n+1} \neq 0$) is called the affine part of $\RP^n$ and the other part isomorphic to $\RP^{n-1}$ (e.g. $x_{n+1} = 0$) is called the part at infinity. So in case of $\RP^1$ we have a point at infinity, in case of $\RP^2$ there is an entire projective line at infinity. The following picture shows the decomposition of $\RP^2$ into parts $\R^2$ (the red $x_3 = 1$ plane) and the line at infinity $\RP^1$ corresponding to the lines in the green $x_3 = 0$ plane. Of course, the $x_3 = 0$ plane may itself be decomposed into an affine part, the dark blue line $x_2 = 1$ (and $x_3 = 0$) and the point at infinity represented by the light blue line $x_2 = 0$ (and $x_3 = 0$).
Remarks.
- To introduce an affine coordinate on a projective space we may choose an arbitrary linear form $\ell(x)=\sum_{i=1}^{n+1} a_i x_i$ on $V$ and decompose the points of the projective space depending on whether $\ell(x) = 0$ or $\ell(x) \neq 0$. In the decomposition above the linear form is $\ell(x) = x_{n+1}$.
- A projective space should be thought of as a “homogeneous object” (to be made precise). So there are no preferred directions (points of projective space) as the hemisphere model or the introduction of an affine coordinate might suggest.
- The same definitions are used in the complex case and yield complex projective spaces: $\CP^1 = \C \cup \{\infty\} = \hat\C$ is the Riemann sphere or complex projective line.
Definition. A projective subspace of the projective space $P(V)$ is a projective space $P(U)$ where $U$ is a vector subspace of $V$. If the dimension of $P(U)$ is $k$ (the corresponding vector subspace has dimension $k+1$), the we call $P(U)$ a $k$-plane.
A $0$-plane is a point, a $1$-plane a line, a $2$-plane a plane, and if $P(V)$ has dimension $n$ a $(n-1)$-plane is a hyperplane.
Proposition 2.1. Through any two distinct points in a projective space there passes a unique projective line.
Proposition 2.2. In a projective plane two distinct projective lines intersect in a unique point.
These two propositions can be proved using linear algebra. Further properties of vector subspaces may be transferred to properties of projective subspaces:
- The intersection of two projective subspaces $P(U_1)$ and $P(U_2)$ is $P(U_1) \cap P(U_2) = P(U_1 \cap U_2)$.
- The projective span or join of two projective subspaces $P(U_1)$ and $P(U_2)$ is $P(U_1 + U_2)$.
- From the dimension formula of linear algebra we obtain a dimension formula for projective subspaces:
\begin{align*}
\dim(U_1 + U_2) &= \dim U_1 + \dim U_2 – \dim (U_1 \cap U_2) \\
\dim P(U_1 + U_2) &= \dim P(U_1) + \dim P(U_2) – \dim P(U_1) \cap P(U_2)
\end{align*}