Pole-Polar Relationship

• b is non-degenerate symmetic bilinear form (e.g.scalar product on $\mathbb{R}^n$)
• orthogonal complement of a vector subspace $U\subseteq V$
  \[U^{\perp}= \left\lbrace v\in V\mid b\left(u,v\right) = 0,\forall u\in U\right\rbrace \]
• $dimU^{\perp} + dim U = dim V$, but $U^{\perp} \oplus U \neq V$.
• If $P\left( U\right)$ is a projective subspace of $P\left(V\right)$,then $P\left(U^{\perp}\right)$ yields another subspace of $P\left(V\right)$.
• in $\RP^2 = P(\mathbb{R}^3)$ we obtain:
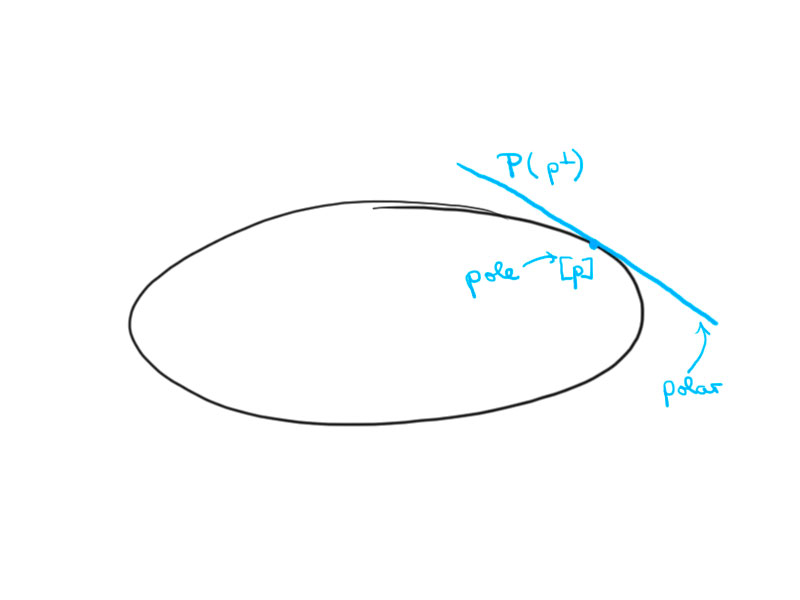
  Point $\left[ P\right] \leftrightarrow$ line $P\left( \left\lbrace p\right\rbrace ^{\perp}\right)$
  Line $P\left( U\right)\leftrightarrow$ point $P\left(U^{\perp}\right)$

Proposition (last lecture).

Proposition. Let $P_1,P_2 \in \RP^2$ and $\ell$ non-degenerate conic, then the polar lines of $P_1$and $P_2$ intersect in the pole of the line $P_1P_2$.

Proof. Let $P_1=\left[ p_1\right], P_2 = \left[p_2\right]$ and $P = \left[p\right]$. Then we have $b\left(p_1, p\right)=0$ and $b\left (p_2, p \right )=0$, since $P \in P\left(p_1^{\perp}\right) \cap P\left(p_2^\perp\right)$. Thus:
\[
b(\lambda p_1+\mu p_2, p)=0,\forall \lambda ,\mu \in \mathbb{R}.
\]

So the line $P_1P_2$ is the polar line of $P$.

Construction of Polar Line for points “outside” and “inside” the conic section.

• $P$ is outside the conic, then the polar line can be constructed using the two tangents touching the conic as shown in the picture.
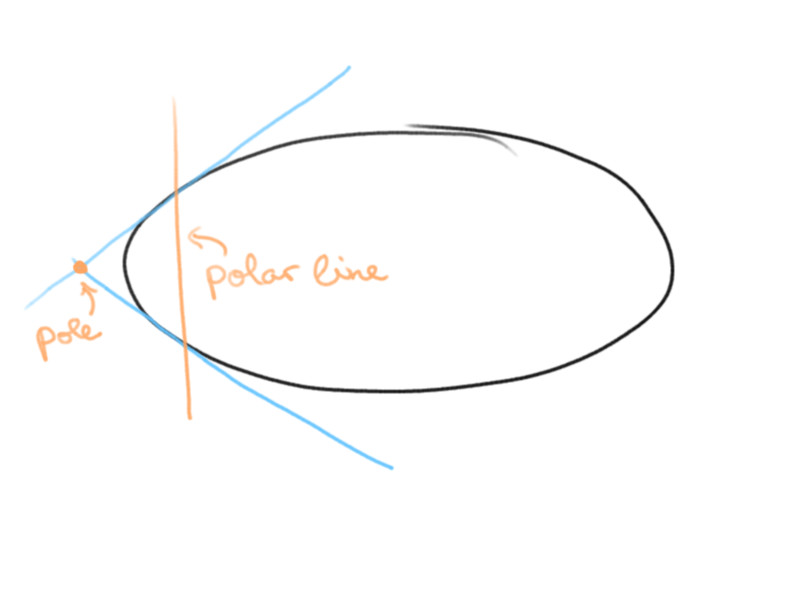
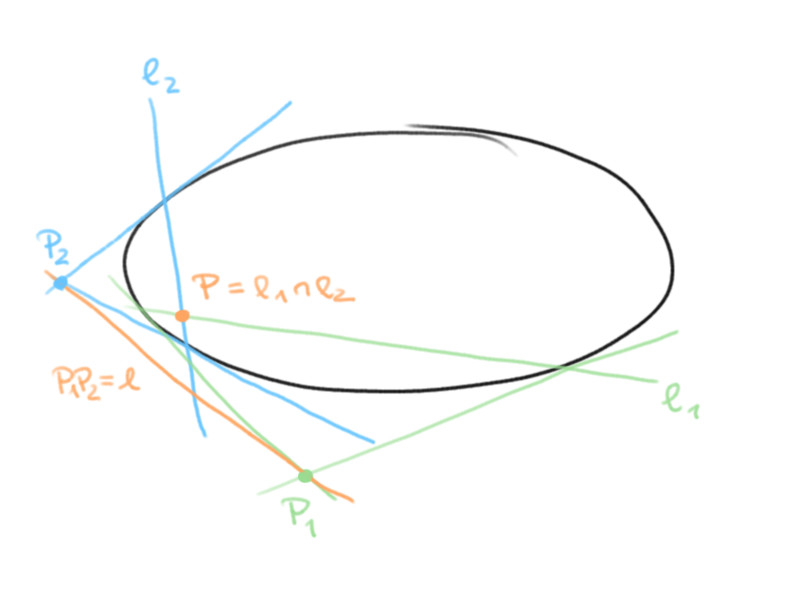
• $P$ is inside the conic, then we consider two arbitrary lines $\ell_1$ and $\ell_2$ through $P$. The polar line $\ell$ of $P$ is the line through $P_1$ and $P_2$ that are the poles of $\ell_1$ and $\ell_2$.

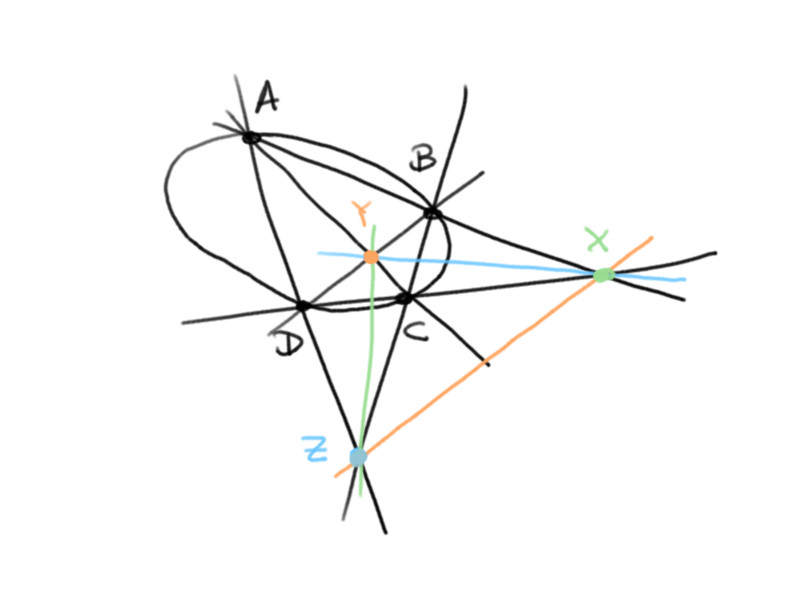
Theorem (Polar Triangle). Let $A,B,C,D$ be four points in general position on a non-degenerate conic in $\RP^2 $,then the intersection points of pairs of opposite sides of the complete quadrangle$A,B,C,D$ form a polar triangle.
i.e.
\begin{align*}
X \text{ is the pole of } YZ\\
Y \text{ is the pole of } XZ\\
Z \text{ is the pole of } XY\\
\end{align*}

If we choose the basis $B =\left\lbrace x,y,z\right\rbrace$ with $X=\left[x\right],Y=\left[y\right],Z=\left[z\right]$,then the above is equivalent to the matrix of bilinear form being diagonal. Additionally, the statement holds for arbitrary non-degenerate conics in the pencil through $A,B,C,D$.

Proof. Normalize:

\[
A= \begin{pmatrix}-1\\1\\1\end{pmatrix}, \;
B= \begin{pmatrix}1\\1\\1\end{pmatrix}, \;
C= \begin{pmatrix}1\\-1\\1\end{pmatrix}, \;
D= \begin{pmatrix}-1\\-1\\1\end{pmatrix}.
\]
Then
\[
X= \begin{pmatrix}1\\0\\0\end{pmatrix},\;
Y= \begin{pmatrix}0\\0\\1\end{pmatrix},\;
Z= \begin{pmatrix}0\\1\\0\end{pmatrix}.
\]
If
\[
B= \begin{pmatrix}
b_{11} & b_{12} & b_{13}\\
b_{12} & b_{22} & b_{23}\\
b_{13} & b_{23} & b_{33}
\end{pmatrix}
\]
then we obtain by evaluating the bilinear form on the points

1. For $A$ on the conic,we get \begin{equation}b_{11}+b_{22}+b_{33}-2b_{12}-2b_{13}+2b_{23}=0\end{equation}
2. For $B$ on the conic,we get \begin{equation} b_{11}+b_{22}+b_{33}+2b_{12}+2b_{13}+2b_{23}=0 \end{equation}
3. For $C$ on the conic,we get \begin{equation}b_{11}+b_{22}+b_{33}-2b_{12}+2b_{13}-2b_{23}=0\end{equation}
4. For $D$ on the conic,we get \begin{equation}b_{11}+b_{22}+b_{33}+2b_{12}-2b_{13}-2b_{23}=0\end{equation}

$$(1)-(2):\ \ \ -4b_{12}-4b_{13}=0\Rightarrow b_{12}+b_{13}=0$$
$$(1)-(3):\ \ \ -4b_{13}-4b_{23}=0\Rightarrow b_{13}+b_{23}=0$$
$$(1)-(4):\ \ \ -4b_{12}-4b_{23}=0\Rightarrow b_{12}+b_{23}=0$$
$\Rightarrow b_{12}=b_{13}=b_{23}$
$\Rightarrow B= \begin{pmatrix}
b_{11} & 0 & 0 \\
0 & b_{22} & 0\\
0&0 & b_{33}
\end{pmatrix}$
$$(1)+(2)+(3)+(4):\ \ \ \ \ \ b_{11}+b_{22}+b_{33}=0$$ so $B$ w.r.t basis$\left\lbrace X,Y,Z\right\rbrace $ is diagonal.

In the above calculation we only used that the points $A$, $B$, $C$, and $D$ are on the conic. So $X$, $Y$, $Z$ is a polar triangle for an arbitrary non-degenerate conic in the pencil. The pencil can be generated by
\[
B_1 =
\begin{pmatrix}
-1 & 0 & 0 \\
0 & 1 & 0\\
0&0 & 0
\end{pmatrix} \text{ and }
B_2 =
\begin{pmatrix}
0& 0 & 0 \\
0 & -1 & 0\\
0&0 & 1
\end{pmatrix}
\]

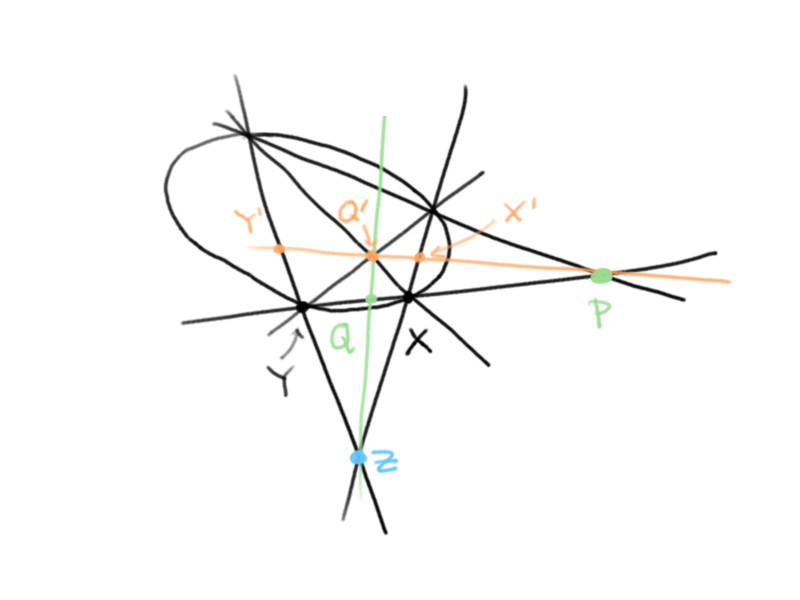
Corollary. Consider a non-degenerate conic $\mathcal{C}$, a point $P$ not on $\mathcal{C}$ and a line $\ell$ through $P$ intersection $\mathcal{C}$ in two points $X$ and $Y$.Let $Q$ be the intersection of $\ell$ with the polar line of $P$, then
\[cr\left(P,X,Q,Y\right)=1\]

Proof. Use the theorem on the polar triangle and the theorem on the complete quadrilateral to obtain:

\[ \cr(P,X’,Q’,Y’) = -1.\]

Then a central projection with center $Z$ yields the desired result.

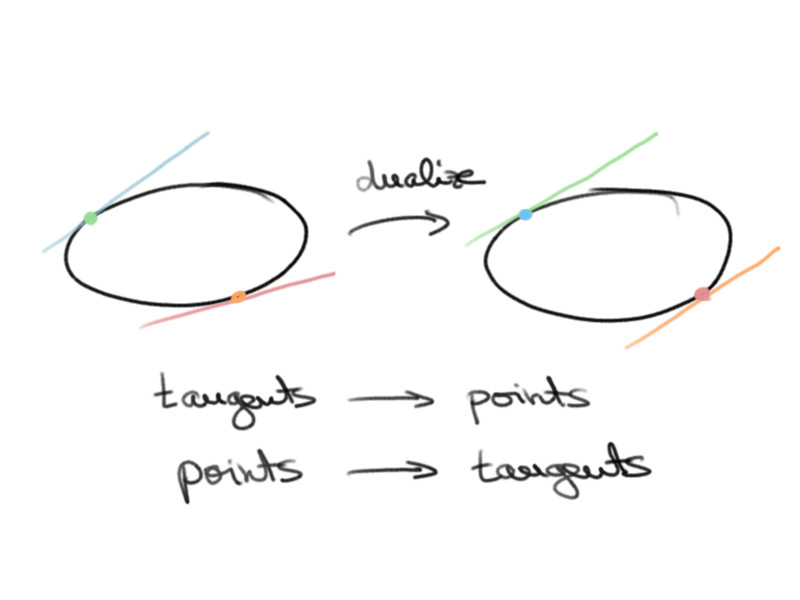
Dual conics and Brianchon’s Theorem

Let $\mathcal{C}$ be a non-degenerate non-empty conic in $\mathbb{R}P^2$.

Then the set of tangents to $\mathcal{C}$ yields a conic in $\left(\RP^2\right)^*$.(homework)

Brianchon’s Theorem. Let $A,B,C,D,E,F$ be a hexagen cirumscribed around the a conic (i.e.\ $AB,BC,\ldots$ are tangents),then the diagonals $AD,BE,CF$ intersect in one point.

Proof. Dualize it and then use Pascal’s theorem.

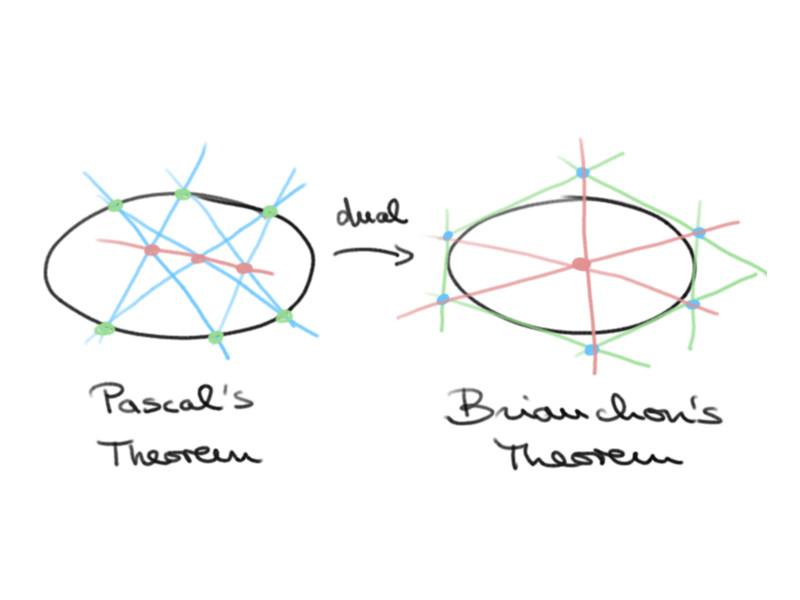Note that the cyclic order of the points on the conic is preserved. In the above picture this is not the case! The order of the points/tangents was intentionally changed to obtain a nice picture for both of the theorems.

Use your favourite interactive geometry software to study what happens when you change the order of the points in the hexagons.